Challenge 13 – The Last Total Solar Eclipse on Earth
Over the span of millions of years, the geometric relationship between the sun and moon that allows total solar eclipses must inevitably change. Although the diameters of our sun and moon have not changed very much in the last few billion years, there have been changes in the distances between them and our Earth. We have total solar eclipses because, given the current distances and diameters, the sun and moon can have nearly the same apparent angular sizes as viewed from the surface of Earth. This allows the disk of the moon to completely cover the far more distance and larger disk of the sun.
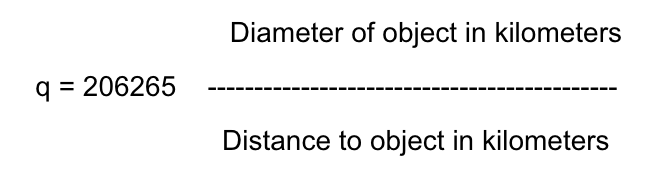
The angular diameter of an object in the sky can be described by the following formula:
The answer will be in angular units called arcseconds. There are 3,600 arcseconds in one angular degree.
There are four physical measurements that determine the apparent sizes of the sun and moon
- The moon’s physical diameter, which is currently 3,474 km.
- The sun’s physical diameter, which is currently 1,392,000 km
- The distance between the moon and the surface of Earth.
- The distance between the center of the Earth and the center of the sun.
Problem 1 – If the average distance to the sun is 149,000,000 kilometers, what is the angular diameter of the sun as viewed from Earth in A) arcseconds and B) degrees.
The moons diameter has not changed during the last 4 billion years, so we can stop worrying about how it affects eclipses in the future. The other three factors, however, all change over time measured in millions or billions of years.
The sun is a star that fuses hydrogen to helium in its core. It has been doing this since soon after its formation about 4.5 billion years ago. As it steadily depletes its hydrogen fuel and builds up helium ash in its core, its core steadily gets hotter and this causes the outer surface to slowly expand. Between 2 billion years ago and 6.5 billion years from now, this slow change in diameter can be physically modeled using sophisticated computer models. A simple formula gives the diameter of our sun as a function of its age in billions of years:
Diameter = 1,392,000 (0.0073 T2 -0.028 T +0.98) kilometers.
Problem 2 – Show that for today at an age of 4.5 billion years, the formula gives the current diameter.
Problem 3 – Convert this formula into one that gives the angular diameter of the sun as viewed from the Earth in arcseconds.
The orbit of our moon has changed significantly over the past billions of years. The tidal gravity force that the Earth produces deforms the moon. The moon does likewise to Earth but energy is lost through friction, and so to conserve angular momentum, the Earth and moon must rotate slower, and their distances have to grow larger over time. This drifting outwards of the lunar orbit has been measured using Apollo laser reflectors left on the moon in the late-1960s, and amounts to 3.8 centimeters per year!
Problem 4 – Closest distance between Earth and Moon occurs at perigee, and is about
356,400 km. Create a formula that predicts the perigee distance to the moon with T measured in billions of years.
Problem 5 – Write the formula for the angular size of the moon in arcseconds.
Now, the orbit of our Earth also changes in time, but these changes are very small over billions of years. As our sun emits a wind of particles into space and converts mass into radiation, the gravitational effect of this miniscule mass loss on Earth’s orbit is not more than about 200,000 kilometers over 10 billion years. This is insignificant compared to Earth’s average distance of 149 million kilometers, so we will not consider changes to the Earth-sun distance.
So we now have two equations that account for the major changes in the angular sizes of the moon and sun as viewed from Earth. We can now ask the question: during what period of time will the lunar diameter be larger than the solar diameter so that the moon produces a total solar eclipse?
Problem 6 – Graph these two functions and find the age of our solar system when the angular diameter of our moon becomes less than that of the sun. How many years into the future is this if the current age of Earth is 4.5 billion years?