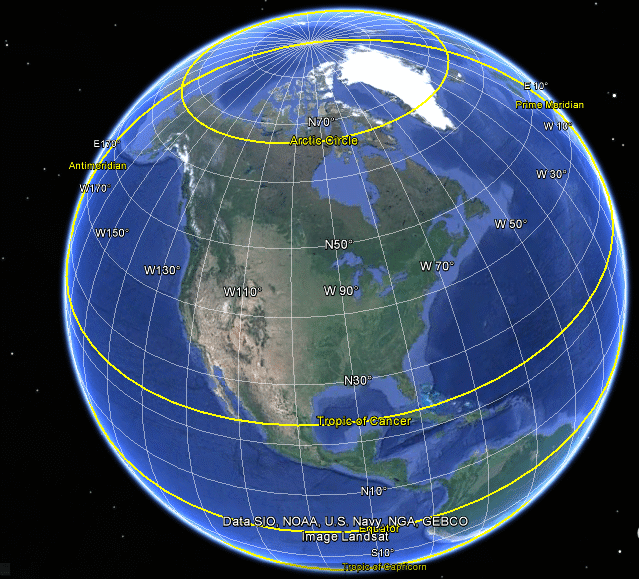
Challenge 1 – Working with Geographic Coordinates
This figure shows the geographic latitude and longitude grid covering North America. In the Northern Hemisphere, latitudes increase from the Equator to the North Geographic Pole, and are measured in angular degrees, minutes and seconds. All circles with the same latitude are concentric with the NGP as shown in the figure. Longitude is also measured in degrees, minutes and seconds and across North America, circles of longitude, called meridians, intersect the NGP and increase from 70o West over Maine, to 125o West over the Pacific Coast.
Credit: NOAA, US Navy, Landsat
The meridians of longitude are also related to what your wall clock indicates as the time of day. Because Earth rotates from the west to the east, as you move in longitude from the East Coast to the West Coast, the sun will rise earlier and earlier in local time.
We all know that a full circle represents 360 degrees, but we can also divide degrees into even smaller measures of angle. The first of these is the arcminute. There are 60 arcminutes in one degree, which we abbreviate by saying that every degree can be divided into 60 minutes. Each arcminute can also be divided into 60 arcseconds, so a full degree has 60 minutes and also 60x60=3600 seconds of arc. We can represent angular measure by a decimal number like 56.5o, or we can write it in the following form: Degrees : Minutes : Seconds. For example 56.5o can also be written as 56o:30’:00”.
When you are given a decimal angle, you can convert it into the D:M:S format. Here is a worked example:
Decimal: 56.398o
Degrees = 56o
Minutes = (56.398 – 56)*60 = 23.88 so M = 23’
Seconds: (23.88 – 23)*60 = 52.8”
So we get: 56o:23’:52.8” or just 56:23:52.8 where the measures are understood.
Problem 1: Convert the following geographic coordinates into the complementary form.
- New York City, Statue of Liberty: 74:02:40.74 West 40:41:21.5 North
- Kennedy Space Center: 80:40:54.2 West 28:31:24.6 North
- Golden Gate Bridge: 122.4783 West 37.8188 North
- Chaco Canyon Ruins: 107.9561 West 36:05:53 North
Problem 2: Convert the following angular measures into the standard d:m:s format.
- Latitude 127,695.5”
- Longitude 6,915.5333 ’
Problem 3: A bicycler is setting up a series of way stations along her journey from Washington, D.C to Portland Oregon. If Washington DC is located at latitude 38o : 54’ 18” North and longitude 77o : 02’: 13” West, and Portland is at latitude 45o : 32’ : 38” North and longitude 122o : 39’ : 15” West, what will be the location of the mid-way point?
Geographic coordinates are good for locating a spot on Earth, which is a spherical planet, but what we also want to know is how far apart different spots are. This is easy to do if the spots are along the same meridian because the circumference of all meridian ‘great circles’ is exactly the same, namely, the circumference of the Earth, which is 40,000 kilometers. If they are at the same latitude but different longitudes, a full 360-degree circle has a circumference less than 40,000 kilometers, so there are fewer kilometers per degree than along a meridian circle!
How far apart are they in kilometers?
- How many degrees apart in latitude are these locations?”
- How far apart are they in kilometers?’
Problem 5: A traveler wants to drive across Kansas on Highway 70 from Kansas City at longitude 94o : 38’ : 14” West to Goodland, Kansas located at longitude 101o : 42’ : 40” West.
- How many degrees apart in longitude are these locations?”
- At the latitude of Kansas City, 39o North, one degree of longitude equals 86 kilometers. How far apart are they in kilometers?’
- If a traveler in Northern Canada at a latitude of 70 degrees traveled between the same longitudes as our Kansas driver, what would be the distance if at this latitude 1 degree in latitude corresponded to a distance of 38 kilometers?